Monopolium
Magnetic monopoles possess units of elementary magnetic charge: that is, a north magnetic charge or a south magnetic charge. An "atom" of a magnetic monopole with captured protons (and perhaps electrons as well) is called monopolium (pronounced: mon-o-po-li-um). Originally a prediction of various (then unconfirmed) Grand Unified Theories (GUTs) on 20th century Earth, monopolium was discovered in abundance in the Iota Persei star system. The discovery of monopolium led to the emergence of many new technologies, largely in virtue of portable room temperature fusion power generation, using 3He nuclei. Perhaps the most important of these are high-energy graviton technologies, which allow for comfortable one-g habitation across the entire Iota Persei star system. Sadly, gravity control does not allow for faster than light (FTL) or hyperfast interstellar travel.
Monopolium Properties
Unlike elementary particles (e.g., electrons), magnetic monopoles are not excitations of a "monopole" quantum field. Rather, they are topological solitons, which are localized regions of spacetime where a higher symmetry between fundamental forces remains compared to the rest of the universe. So, a GUT-scale magnetic monopole has a core region where the strong nuclear and electroweak forces are still unified. So during the Big Bang when the strong force split off from the electroweak force, the universe underwent a "phase transition" similar to how water becomes ice. Magnetic monopoles are defects in the "freezing out" of the universe (e.g., "cracks" in the "ice"). It is for this reason and that magnetic charge must be conserved, that magnetic monopoles will never undergo radioactive decay: they are stable over astronomical timescales.
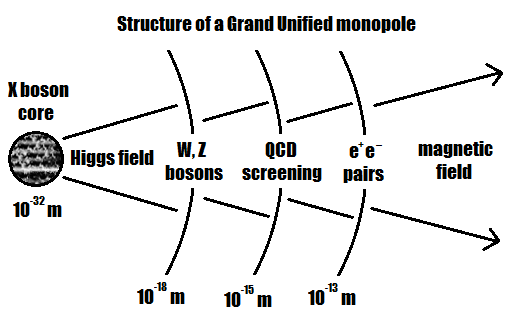
There is only one known type of magnetic monopoles, corresponding to the only first-order symmetry breaking event that took place during the Big Bang: GUT monopoles corresponding to the Grand Unified Scale at MX ≈ 1016 GeV (far above the Electroweak Scale, a second-order symmetry breaking event, where MW ≈ 102 GeV). Magnetic monopoles have a core of magnetic energy 10-32 m radius. It is important to note that all monopoles have a rather complex structure outside of the core. Within a radius 10-18 m, magnetic charges are anti-screened or vanish and monopoles enter a bound state with one another in virtue of the Higgs Field post-electroweak symmetry breaking (see the diagram below of a monopole's structure). Beyond a radius of 10-13 m monopoles behave like elementary particles: mere magnetic monopoles. The mass of a magnetic monopole is 1.03 x 1018 GeV, only 12 times less than Planck Mass (MP ≈ 1.22 x 1019 GeV or 22 micrograms).
Atomic Monopolium
In virtue of the dipole magnetic moment of elementary particles, some can couple with the magnetic charge of a monopole, and be captured into a bound state analogous to how atomic nuclei can capture electrons to form atoms. Unfortunately, unstable particles like neutrons are not held with sufficient binding energies by a magnetic monopole to make them no longer radioactive. A free neutron undergoes β- decay releasing 782 keV, far more than the binding energy of 10.36 keV. Thus, remaining bound to a magnetic monopole is not energetically favorable for neutrons. Electrons (nor positrons) can be captured by a solitary magnetic monopole into bound states either. This is due to the Schrödinger equation correctly predicting that an electron or positron will always have a repulsive centrifugal force in the presence of magnetic charges (in other words: monopolium is a superinsulator).
Protons however are not only stable around monopoles but they are relatively immune to nuclear fusion catalyzation, since an early step of proton—proton fusion requires a rare intervention by the Weak Force (something a monopole cannot assist with). With a 1s shell binding energy to GUT monopoles of 15.1 keV, monopolium can be extremely dense due to the 1s shell's radius of around 48 fermis. This distance is also far away enough from the monopole to avoid proton decay. However, with the presence of protons at a nuclear distance from the monopole, electrons can be captured at energies such as what happens inside of a hydrogen or helium atom. Now, there is no 2s shell for protons bound to a monopole, since the net angular forces will be repulsive. Hence the maximum number of protons a monopole can capture is merely two, of opposite spin, to fill the proton's only s shell. More than two electrons can be bound to a monopole-proton system, with the whole system (monopole-protons-electrons) behaving as negatively charged ions.
Monopolium Shells
Ionizing Radiation
Magnetic monopoles are highly ionizing (i.e., can strip electrons from atoms, with those electrons producing X-rays as secondary radiation) if encountered as free particles. Thus, they can be quite dangerous for both biological and electronic entities.
Gravitational Radiation
Monopoles are so close to Planck Mass that their excitations produce quantum gravitational effects. It was in the examination of magnetic monopoles that the graviton (the spin-2 boson, G, responsible for gravitational interactions) was finally discovered. GUT-scale monopoles possess a mutual gravitational interaction some 1042 times stronger than the gravitational interaction between two electrons, and have an electromagnetic interaction 4692 times stronger than that between electrons. So, since the electromagnetic force is 1037 times stronger than gravitation, the high energy gravitons produced by a system of magnetic monopoles end up being several times more potent than photons produced by that same system. These high frequency gravitons correspond to extremely strong disturbances in spacetime curvature. If monopoles were less massive by even a few orders of magnitude, the emission of photons would dominate and gravitons would remain unknown.
Since ordinary matter (protons, neutrons, and electrons) is very weakly coupled with the gravitational interaction, monopolium is much better suited for graviton detection devices. Since monopolium is involved with both gravitation and electromagnetism, conversions between the energies of those two fundamental interactions is accomplished in a practical manner with monopolium. That is, if a magnetic monopole is struck by a graviton, there is a good chance that a photon will be emitted, which can then be detected by ordinary matter. If GUT-scale monopoles were much more massive than they are, graviton emissions would dominate and graviton—photon conversion techniques would be impractical.
Investigations into gravitons led to the discovery of not only a slew of quantum gravitational phenomena, but the specifics of the nonlinear nature of gravity in a quantum mechanical way. Some of these nonlinear quantum corrections includes the positing of paradoxical effects among high-energy gravitons: sometimes repulsive gravitational fields appear in highly warped spacetimes. Further research allowed scientists to no longer need various ad hoc corrections to the Einstein Field Equations, with a proper theory of quantum gravity in their possession.
No Hyperfast Travel
Main Article: No Hyperfast Travel
Since the Flux Energy Condition (ρ² ≥ P²) holds in quantum gravity (that is, quantum gravity is consistent with Special Relativity), superluminal flows of energy and momentum are strictly forbidden. This means that faster-than-light or hyperfast travel and communication is entirely unphysical.
Monopolium Technologies
Nuclear Fusion Catalyzation
If two 3He nuclei approach too close to a magnetic monopole, they will overcome their Coulomb repulsion and fuse at room temperature, outgassing only alpha particles and protons. Thus, aneutronic and aphotonic cold fusion becomes possible with magnetic monopoles. Since the outgassed charged particles can induce electric currents to power equipment, this type of fusion technology is extremely energy efficient. The same technology can be used on a much grander scale for clean nuclear fusion rocketry, with an exhaust velocity of 6.8% of lightspeed. Monopoles are not expended or exhausted by this process, however only nuclei with an odd number of nucleons can undergo fusion catalyzation.
Nucleon Decay Catalyzation
GUT monopoles interact with normal matter by inducing nucleon decay (e.g., proton and neutron decay). The proton decays into a positron (e+) and various pions (π0 or π±). Neutral pions decay into two gamma rays (γγ), and since the kinetic energy of the pions is relativistic (0.94c) both photons will move in the direction of the neutral pion (relative to the apparatus). Charged pions (π±) usually decay into a muon and muon-antineutrino, which the muon promptly decays into an electron and an electron-antineutrino. Hence, GUT monopoles can be used (over and over) to catalyze nucleon decay to provide a near 100% mass to energy conversion power systems and rocket engines, exhaust velocity 94% of lightspeed. As a bonus: this is all without needing to store extremely hazardous and expensive antimatter!
To avoid complications with nuclear fusion catalyzation and the Pauli-exclusion principle, even numbered (or bosonic) nuclei are preferred in technological uses of nucleon decay (e.g., 132Xe or Xenon-132 for nucleon decay rocket engines). These nuclei do not interact with a monopole's magnetic field, and will ignore the presence of captured protons by the monopole.
Graviton Technologies
With the development of quantum gravity in the study of high-energy graviton physics in the Iota Persei star system, various technologies emerged which could exploit the nonlinear features of gravity on a small scale, in virtue of high-energy gravitons produced by GUT monopoles. Unlike the paragravity catapult envisioned by Robert L. Forward in 1963, high-energy gravitons allow for the creation of handheld paragravity devices. The list below covers the most notable of these technologies.
Paragravity
Having 6.2 mg/m3 of monopoles allows one to generate a sufficient amount of high-energy gravitons to duplicate a comfortable one-g environment, for use in either microgravity environments (spaceships) or celestial environments (colonial arcologies on moons and planets). Paragravity is essential for most habitation beyond Earth, since the human body has a very small window of tolerance regarding gravity, for long-term health. Since such a small amount is required for Earth-like gravity generation, personal and vehicle paragravity belts are common. The small range of such devices relative to that of Earth's radius and the density of the monopolium compared to that of Earth's density helps to reduce the bulk quantity of monopolium required (by 23 orders of magnitude), especially since the high density increases the presence of nonlinear interactions between gravitons. However, all such devices obey conservation of momentum, and thus cannot be used to generate free kinetic energy.
Stressor Beams
An extension of paragravity technology is the production of a beam of high-energy gravitons that induces intense oscillations in the curvature of spacetime. That is, a beam that induces gravitational stress on anything entering it. Such high frequency spacetime curvature oscillations (near the Planck scale) can induce severe damage to objects (much like acoustic vibrations), and every object is affected equally in virtue of the equivalence principle of General Relativity (which quantum gravity upholds). Such beams drop off at 1/r², thus with a beam divergence in kind with that of flashlights. There is no known method by which to generate a gravitational analogue of a laser at this time. Since gravity is nonlinear, stressor beams do interact with each other, unlike the linear dynamics that obtains for electromagnetic fields.
Natural Occurrence
While relic magnetic monopoles from the Big Bang should exist, none have been found thus far. However another type of topological defect, a cosmic string, appears to have crossed paths with another cosmic string, and produced tremendous numbers of gravitational waves and magnetic monopoles, enriching the Iota Persei star system with monopolium ore.
Cosmic Strings
Cosmic strings are a proposed relic from the Big Bang, a topological defect or soliton like magnetic monopoles are. Magnetic monopoles can be thought of as zero-dimensional defects, and cosmic strings are one-dimensional defects: a very thin object (10-32 m radius) under immense tension, potentially spanning the size of the observable universe. Their proposed mass density is equal to their tension, some 1021 kg per meter of length. If a cosmic string intersects another, they break and form a slew of magnetic monopoles, gravitational waves, and closed loop cosmic strings. These closed loops then continue to decay gravitationally, over astronomical timescales.
It has been speculated that the cosmic occurrence of relic cosmic strings from the Big Bang are around one per billion cubic light-years. Cosmic strings are very hard to locate at a distance, since their tension and mass density cancel out gravitationally (tension is also negative pressure, a component to be calculated in the stress-energy tensor of General Relativity), they exert no gravitational forces on nearby objects despite their immense mass. However, light passing near a cosmic string will encounter a "conical deficit": the area around a cosmic string is less than 360° and will bend light, creating a double image of objects behind a cosmic string.
Monopolium Ore
Bulk monopolium is never found in a pure state: it is usually intermixed with ferromagnetic elements like iron and nickel (usually all three mixed together). It is their magnetic properties which draws in magnetic monopoles from the interstellar medium. This is a negative feedback loop, for as the magnetized iron-nickel attracts more monopoles, any magnetic field is "poisoned" by those monopoles. Monopolium ore appears like any iron-nickel deposit, except that there is the presence of small translucent nodules, the more monopolium rich parts of the iron-nickel ore. Monopolium has a low plasma frequency, and so appears transparent when not mixed with iron-nickel material.
Since pure monopolium is a prerequisite for its technological use, melting monopolium ore is essential. Since the melting point of the various types of monopolium is well over that for all the elements in the periodic table, one can "drain off" the now liquid iron and nickel and be in possession of purified monopolium. Initially, the liberated monopolium exudes from the molten ore as microscopic dust particles, collected by a electromagnetic dust trap (usually coated with tungsten for its high melting point). However, since purified monopolium dust approaches the density of a neutron star (>1018 kg/m3), it is suspended as a gas in a magnetic bottle to reduce the density for transport.
References
General Physics
Original Usenet Physics FAQThe Physics Stack Exchange
Atomic Rockets by Winchell Chung
Forward Paragravity (1963)
Forward Indistinguishable from Magic (1998)
The Flux Energy Condition (2011) / (Free Version)
Magnetic Monopoles
Short Primer on Magnetic Monopoles (Free 2017)Good Primer on Magnetic Monopoles (1984)
Extensive Research on Magnetic Monopoles (1983)
No Electron Bound States to Monopoles (1948)
Electron Bound States to Atomic Monopolium (1951)